
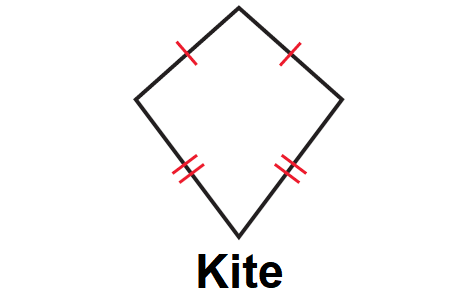
A kite is traditionally defined as a four-sided, flat shape with two pairs of adjacent sides that are equal to each other.
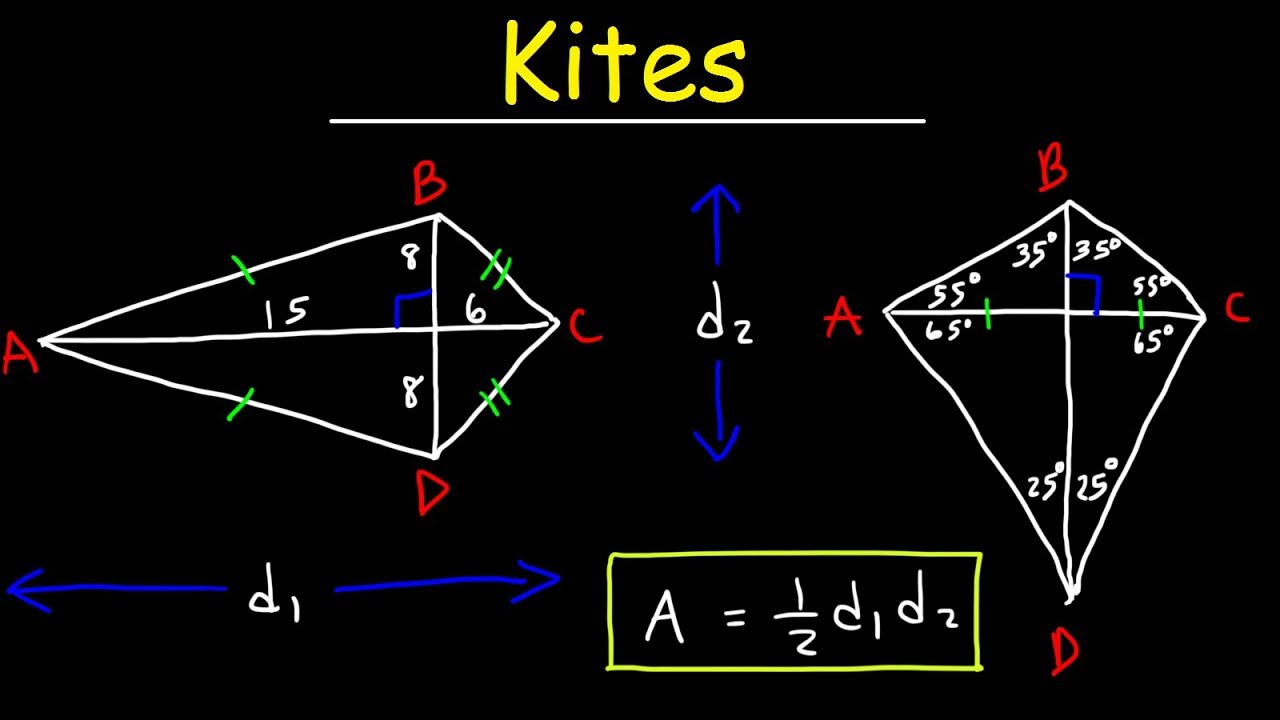
One of the diagonals in a kite bisects its non-congruent angles. This lecture is tutorial study based presentation. Diagonal AC is the perpendicular bisector of diagonal BD.ĭiagonals that bisect the angles of a kite Therefore, diagonals AC and BD are perpendicular. Based on this, we know that line segment from A and C to the midpoint of BD is the heights of △ABD and △CBD. Therefore, △ABD and △CBD are isosceles triangles that share a base, BD. Kites have a couple of properties that will help us identify them from other. Kites also have the following properties: Perpendicular diagonals. Diagonals of a kiteĭiagonals are perpendicular to each other:įor kite ABCD shown above, BA ≅ DA and BC ≅ DC. A kite is a quadrilateral with two pairs of congruent, consecutive sides. Sides AB and BC and sides CD and DA are adjacent non-congruent sides for kite ABCD above, so ∠B≅∠D. It has two pairs of equal sides, and the two sides in each pair are. The angles formed by the adjacent, non-congruent sides of the kite are congruent. In geometry, a kite or deltoid is a type of quadrilateral, a four-sided polygon. Unlike a parallelogram the congruent pairs of sides are not opposite of each other.įor kite ABCD above, congruent sides BC and CD are adjacent to each other as are congruent sides AB and AD. Like a parallelogram, a kite has two pairs of congruent sides. Sides and angles of a kite Sides of a kite You may think of the kite that can fly, like the one below, when you think of the shape of a kite. These equal sides share a vertex, or "corner." By definition, a kite shape may be either convex or concave, but it is often shown only in its convex form. In mathematics, a kite shape is a quadrilateral with two pairs of sides that are of equal length. Kite properties include (1) two pairs of consecutive, congruent sides, (2) congruent non-vertex angles and (3) perpendicular diagonals.


 0 kommentar(er)
0 kommentar(er)
